Background:
For those that may not know, the Bechdel test has 3 criteria. A movie/TV show/book/whatever must have at least 1 scene where there exist:
0. 2 female characters
1. talking to each other
2. about something other than a man
A rather depressing amount of movies/TV shows fail this relatively simple test. Most characters seem to be male, and the female ones exist just so that men will have love interests. Since I’m a numbers guy, I wondered what fraction of media would pass the test if there were actually no sexism.
Parameters:
N = The number of characters
C = The number of distinct conversational pairings in a given item of media. A “conversation pairing” means any 2 characters talking to each other, and distinct means at least 1 of the characters is different than any other pairing.
p_g = Fraction of characters of an acceptable gender (ie female)[1]
p_t = Probability that a conversation is about an acceptable topic (ie something other than a man)
For each conversation that happens, the probability it will cause the item to pass a Bechdel-type test is:
Pr(1 convo passes) = (Probability the convo is between two acceptable people) x (Probability the topic is acceptable) ~= p_g^2 x p_t.
Since we aren’t counting conversations one has with oneself, technically that’s a slight overestimate. In general there will be N choose 2 = N * (N-1) / 2 possible conversations. However, only (p_g x N) choose 2 = (p_g x N)(p_g x N-1)/2 are within a given gender. For N = 10 and p_g = 0.5, this is 20/90 = 2/9, a touch less than p_g^2 = 1/4th. So I’m gonna make this approximation, it’s the least of our troubles.
For an item to pass it only needs 1 passing conversation, that probability is:
Pr(whole item passes) = 1 – (1 – Pr(1 convo passes) )^C
It’s rather difficult to estimate how many distinct conversations might exist. In a 40 minute TV show, figuring a scene lasts 2 minutes, there would be time for 20. Given episodes tend to match characters up together, so one would expect multiple scenes with the same people, reducing that number. There also need to be enough characters for this number of distinct conversations to be possible, but that’s usually not an issue. Even a 6-character show would have 15 possible pairings, and that’s assuming no guest stars.
Guessing at p_t is even more difficult. The simpest assumption would be that all conversations are about another character. However, it’s extremely likely that a conversation between two characters of one gender will be about a character of a different gender, since it’s likely to be romantic. That isn’t sexist, just heteronormative. One might also assume that two characters are talking about some other character not on screen, which would be more likely to be the opposite gender (given that we’ve already taken up two characters to have the convo).
So since I can’t come up with a solid number, let’s explore the parameter space a bit. Here are some plausible (re: made up) numbers for each parameter, and the corresponding odds of passing the test
Graph:
Chart:
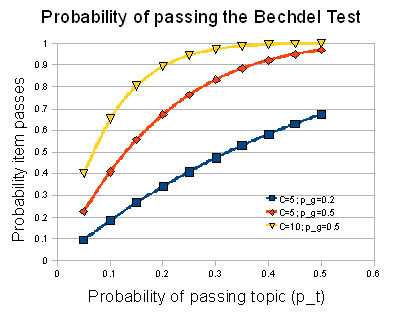
| p_g | C | p_t | Pr(passes) |
| 0.5 | 22 | 0.3 | 1 |
| 0.2 | 5 | 0.05 | 0.1 |
| 0.2 | 5 | 0.1 | 0.18 |
| 0.2 | 5 | 0.15 | 0.27 |
| 0.2 | 5 | 0.2 | 0.34 |
| 0.2 | 5 | 0.25 | 0.41 |
| 0.2 | 5 | 0.3 | 0.47 |
| 0.2 | 5 | 0.35 | 0.53 |
| 0.2 | 5 | 0.4 | 0.58 |
| 0.2 | 5 | 0.45 | 0.63 |
| 0.2 | 5 | 0.5 | 0.67 |
| 0.5 | 5 | 0.05 | 0.23 |
| 0.5 | 5 | 0.1 | 0.41 |
| 0.5 | 5 | 0.15 | 0.56 |
| 0.5 | 5 | 0.2 | 0.67 |
| 0.5 | 5 | 0.25 | 0.76 |
| 0.5 | 5 | 0.3 | 0.83 |
| 0.5 | 5 | 0.35 | 0.88 |
| 0.5 | 5 | 0.4 | 0.92 |
| 0.5 | 5 | 0.45 | 0.95 |
| 0.5 | 5 | 0.5 | 0.97 |
| 0.5 | 10 | 0.05 | 0.4 |
| 0.5 | 10 | 0.05 | 0.4 |
| 0.5 | 10 | 0.1 | 0.65 |
| 0.5 | 10 | 0.15 | 0.8 |
| 0.5 | 10 | 0.2 | 0.89 |
| 0.5 | 10 | 0.25 | 0.94 |
| 0.5 | 10 | 0.3 | 0.97 |
| 0.5 | 10 | 0.35 | 0.99 |
| 0.5 | 10 | 0.4 | 0.99 |
| 0.5 | 10 | 0.45 | 1 |
| 0.5 | 10 | 0.5 | 1 |
The first row is my guess at a realistic value for C, and idealistic values for p_g and p_t. Since it’s a rounding-error from 1.0, I tweaked the numbers to see some variability. We see that in the sexism-free condition (p_g = 0.5), for even a modestly large number of distinct conversation pairings, the probability of passing pretty rapidly approaches 1.
So keep on the lookout. Because until damn near everything you watch passes the Bechdel test, sexism still exists.
-Jacob
- [1]For simplicity of language I’m going to assume that all characters’ gender is equal to their biological sex, and that all characters are either male or female.↩


Thanks for posting this! When I explain the Bedchel test, the most common refutal I get is “what is the null hypothesis?” And this provides the exact data I’m interested in.
Pingback: More on the Bechdel Test | Jacob Silterra